Description
What our Pythagorean Theorem: Advanced lesson plan includes
Lesson Objectives and Overview: Pythagorean Theorem: Advanced teaches students about the Pythagorean theorem and how to use it to solve math problems. At the end of the lesson, students will be able to understand and apply the Pythagorean theorem to solve problems. This lesson is for students in 6th grade.
Classroom Procedure
Every lesson plan provides you with a classroom procedure page that outlines a step-by-step guide to follow. You do not have to follow the guide exactly. The guide helps you organize the lesson and details when to hand out worksheets. It also lists information in the green box that you might find useful. You will find the lesson objectives, state standards, and number of class sessions the lesson should take to complete in this area. In addition, it describes the supplies you will need as well as what and how you need to prepare beforehand.
Options for Lesson
Included with this lesson is an “Options for Lesson” section that lists a number of suggestions for activities to add to the lesson or substitutions for the ones already in the lesson. One optional addition to this lesson is to have your students cut paper triangles out, measure the lengths, and solve for the hypotenuse. If you want your students to practice their measurement skills, you can have them experiment with various lengths of rope and numbers of knots.
Teacher Notes
The teacher notes page includes a paragraph with additional guidelines and things to think about as you begin to plan your lesson. This page also includes lines that you can use to add your own notes as you’re preparing for this lesson.
PYTHAGOREAN THEOREM LESSON PLAN CONTENT PAGES
Pythagorean Theorem
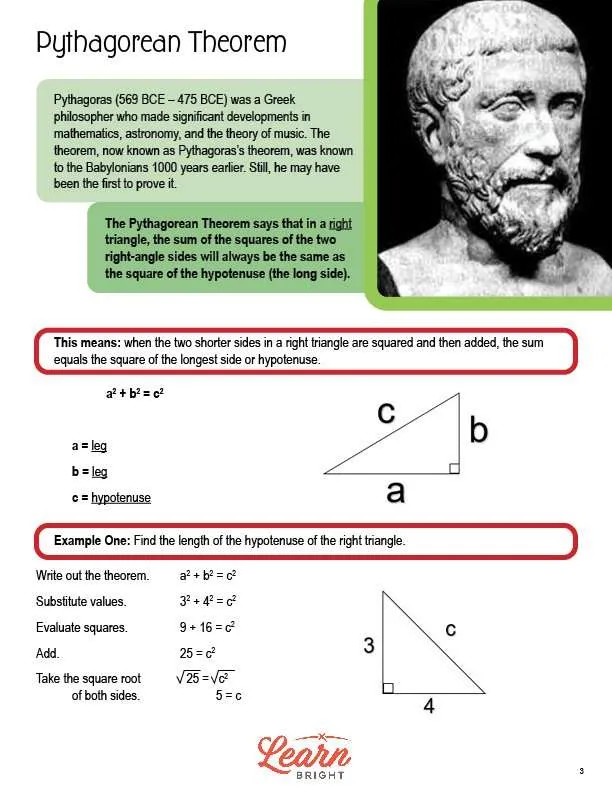
The Pythagorean Theorem lesson plan includes two content pages. Pythagoras (569 BCE – 475 BCE) was a Greek philosopher and mathematician. He contributed greatly to the fields of mathematics, astronomy, and the theory of music. He developed a mathematical theorem (which the Babylonians may have already proved 1,000 years earlier) that we still use today to find the length of a side of a right triangle.
The Pythagorean Theorem says that in a right triangle, the sum of the squares of the two right-angle sides will always be the same as the square of the hypotenuse (the long side). Basically, when you square the two shorter sides of a right triangle and add them, the sum equals the square of the longest side (the hypotenuse).
The Pythagorean Theorem is a² + b² = c². The variables a and b are called the legs, while the variable c is called the hypotenuse.
Let’s take a look at a few examples. For the first example, a = 3, b = 4, and we have no value for c. To solve, first write out the theorem: a² + b² = c². Next, substitute your variables: 3² + 4² = c². Then, evaluate squares: 9 + 16 = c². Next, add: 25 = c². Finally, take the square root of both sides: √25 = √c². Taking the square root of both sides gives us 5 = c, so the length of the hypotenuse is 5 units.
For the second example, a = 6, b = 7, and we have no value for c. Like the first example, to solve, first write out the theorem: a² + b² = c². Next, substitute your variables: 6² + 7² = c². Then, evaluate squares: 36 + 49 = c². Next, add: 85 = c². Finally, take the square root of both sides: √85 = √c². Taking the square root of both sides gives us 9.22 = c, so the length of the hypotenuse is 9.22 units. Remember that not every answer will be whole numbers, but all answers will always be positive.
You may be asking yourself how to identify right triangles with only their measurements. You know that if a² + b² = c², then a triangle is a right triangle. To check whether a triangle is a right triangle, all you have to do is substitute the numbers and solve. When substituting, remember that the largest number is always the hypotenuse.
Let’s look at an example. Do the measurements 9, 12, and 15 make a right triangle? First, write out the theorem: a² + b² = c². Next, substitute: 9² + 12² = 15². Finally, solve: 81 + 144 = 225. This is true, so the measurements 9, 12, and 15 do make a right triangle!
Let’s look at another example. Do the measurements 6, 12, and 7 make a right triangle? First, write out the theorem: a² + b² = c². Next, substitute: 6² + 7² = 12². Finally, solve: 36 + 49 = 144. This is not true (36 + 49 = 85, not 144) so the measurements 6, 12, and 7 do not make a right triangle!
PYTHAGOREAN THEOREM LESSON PLAN WORKSHEETS
The Pythagorean Theorem lesson plan includes three worksheets: an activity worksheet, a practice worksheet, and a homework assignment. You can refer to the guide on the classroom procedure page to determine when to hand out each worksheet.
EGYPTIAN ROPE STRETCHING ACTIVITY WORKSHEET
Students will work in groups to complete the lesson activity. Each group will use a rope with 13 knots tied at equal intervals to create different triangles. They will also answer several questions about the rope and its uses.
MISSING LENGTHS PRACTICE WORKSHEET
For the practice worksheet, students will find the lengths of the missing sides of three triangles, rounding their answers to the nearest tenth if necessary. They will also solve two word problems that require them to use the Pythagorean Theorem.
PYTHAGOREAN THEOREM HOMEWORK ASSIGNMENT
The homework assignment asks students to solve two word problems. For each, they will include a drawing and the math they used.
Worksheet Answer Keys
This lesson plan includes answer keys for the activity worksheet, the practice worksheet, and the homework assignment. If you choose to administer the lesson pages to your students via PDF, you will need to save a new file that omits these pages. Otherwise, you can simply print out the applicable pages and keep these as reference for yourself when grading assignments.